Blog

Will This Be on the Test? (November 2022)

by Sarah Lonberg-Lew

---

Welcome to the latest installment of our monthly series, “Will This Be on the Test?” Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually.

---

Welcome back to our continuing exploration of how to bring real conceptual reasoning to questions students might encounter on a standardized test. Here is this month’s problem:

If you saw this question in a textbook, you might see it followed by an instruction to write and solve an equation to find the answer. And this question can be answered in that way, but there are other approaches that will work and that involve just as much algebraic thinking, although they may involve less algebraic notation. Writing and solving equations using symbols and steps is an abstract way of recording reasoning, but students who are not ready to work with abstract symbols at that level are still capable of reasoning algebraically using more concrete and visual tools.

Here are some possible approaches:

1. Estimate! You can get a handle on almost any math task that has a real world context (and many that don’t) with estimation. In this case, a student might reason that each pen is unlikely to cost as much as $5 or $6 if the whole purchase was under $20. The numbers in this task are small, so computing with them is not too cumbersome, but it is still worth taking some time to decide what a reasonable answer might look like for two reasons: a) if you make a computation or strategy misstep that leads you to an unreasonable answer, having estimated will prompt you to check your work, and b) the process of estimating helps you get a handle on the structure of the situation, setting you up for more precise analysis.

2. Work down to the cost of the pens. Starting with the broadest piece of information in the story, a student might bring in the information piece by piece to get to the information they are looking for, making deductions along the way. Receiving $6 change from $20 means that Ray paid $14 for the notepads and pens. The notepads cost $4 each, so they account for $8 of the amount paid and the rest was for the pens. What might the student do next? Don’t forget that there were 3 pens!

Some students might think of this kind of reasoning as “cheating” because it doesn’t look like the steps you would find in a math book. Students who solve things “their own way” may discount the value of their reasoning especially if their past experience in math class or with math books involved being told to do problems a specific way and to show their work to prove they did it that way. Listening to and validating students’ reasoning as real mathematical thinking is an important part of undoing the damage they may have incurred in math class.

3. Draw a bar model. When a story problem tells how quantities are related, drawing a picture that illustrates that relationship can help a student figure out what operations will get them to the missing information. This model shows that the notepads and pens came to $6 less than the $20 bill:

And this alternative model shows how the $20 bill was broken up.

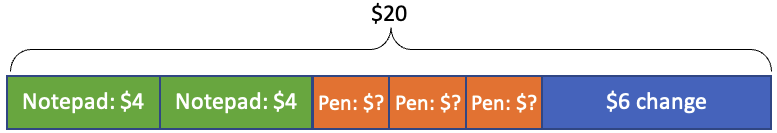
How might these models help a student figure out the cost of a pen? Note that drawing and using bar models takes some practice. It can be helpful to start out providing the models for students so they can learn to reason with them, which will motivate them to draw their own models. Watch this video for an example of students using bar models (which are sometimes called Singapore Strips).

4. Write and solve an equation. As stated at the beginning, this is a problem that can be solved using an equation. In fact, students who have practiced algebraic reasoning enough to be comfortable shortening their notation, may find it quite natural to represent the relationships in the problem using symbols. Here’s one equation a student might write and solve:

2(4) + 3x = 20 ⎻ 6

Look at the first bar model above. How does this equation compare to that representation? How do the steps you might take to solve this equation compare to what you might do to solve the problem with the bar model?

Here’s another possible equation a student could use:

2(4) + 3x + 6 = 20

How does this compare to the second bar model?

Are there other ways you could write an equation for this problem? Could those equations also be represented visually with bar models?

Teachers and students are often in a rush to get to algebra because being able to solve equations by manipulating symbols is what many of us have been taught to believe is what being successful at math looks like. However, writing and solving equations is only a very small part of what algebra really is. Reasoning about quantities and their relationships and using clues to find missing values are skills students can be practicing at all levels and even using to answer algebra questions on tests. As students continue to grow their algebraic reasoning skills, symbolic manipulation will emerge as a solution to an intellectual need for a concise and consistent way to record their reasoning.

---