Will This Be on the Test? (June 2022)
by Sarah Lonberg-Lew
Welcome to the latest installment of our monthly series, “Will This Be on the Test?” Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually.
Welcome back to our continuing exploration of how to bring real conceptual reasoning to questions students might encounter on a standardized test. In April, we looked at a question that mixed contextualized and decontextualized representations of a relationship. Sometimes, though, we encounter questions that are purely decontextualized:
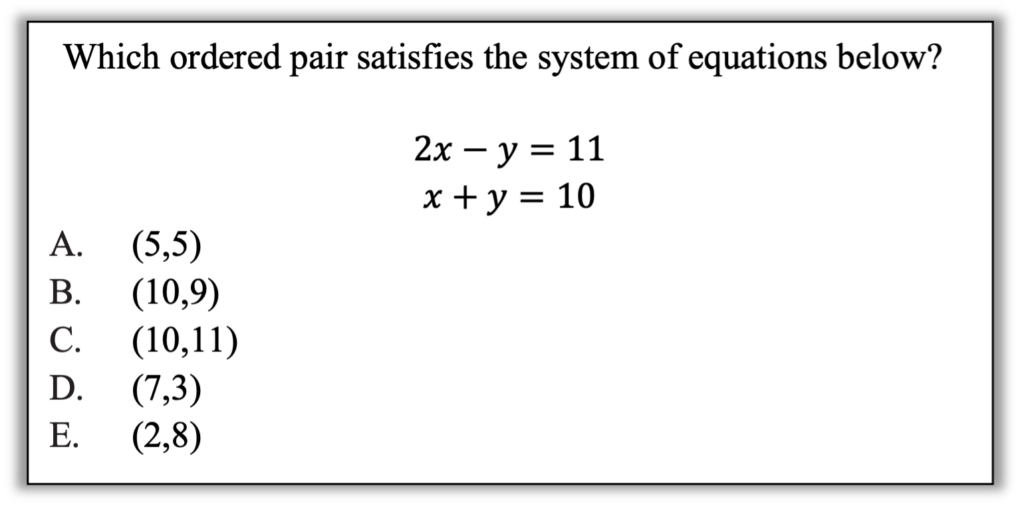

How many ways can you think of to approach this task? Do you have a memorized procedure? How would you tackle this if you didn’t? What skills and understandings do students really need to be able to answer this question? How could you use visuals to help you reason about this?
As I’ve said earlier in this series, not every problem is one for every student to take on. This one is very abstract and for students who are not ready to work with these kinds of relationships at this level of abstraction, it may be best to just make a guess and move on. The progression from concrete to representational to abstract is real and important. Just because a student can reason about a certain algebraic concept concretely does not necessarily mean they are prepared to reason about it abstractly. However, we can help students build that bridge and bring their reasoning to more abstract levels. (By the way, please don’t confuse “abstract” with “high level”. The progression of concrete to representational to abstract is one that happens over and over with each new concept and at every level. Students reasoning more concretely can still take on problems involving systems of equations. See the December 2020 post in this series for an example.)
Also, there are a couple of notational/vocabulary details students need to be comfortable with to solve this problem. Specifically, students need to know what it means for a value to “satisfy” an equation (the equation will be true when the variable is replaced with that value), and they need to understand that the ordered pairs in the answer choices give the values of x and y in that order. These are understandings that can be taught as when they become relevant. When students are ready to abstract their thinking, they will have an intellectual need for these details.
With these understandings, let’s press on to making sense of the problem and persevering in solving it. Here are some possible approaches:
1. Turn it into a story. It could be quite a bit of work to come up with a realistic story that the system of equations models, but a student can still take something that looks like alphabet soup and make it more accessible by telling the story in words. For example, a student might retell the story of the system this way:
There are two numbers. One thing I know about them is that if you double one of them and subtract the other from it, you’ll get 11. The other thing I know is that together they add up to 10. I want to figure out what the two numbers are.
This is probably not the most compelling story you’ve ever read, but it is a way of making sense of the problem that makes it feel more accessible. Framing this “problem” as a game or a puzzle, even a quest, can free a student from feeling stuck trying to remember the right procedure. What ideas about playing with the numbers come to you from reading this story that you might not think of when just looking at the equations?
2. Draw a picture. It can be challenging to draw pictures of subtraction, but the visualizing the relationship in the second equation could really help students get a handle on what kinds of numbers might be solutions to the system. A student who has had opportunities to work with concrete manipulatives like counters, might imagine the second relationship as a group of ten counters in two different colors like this:
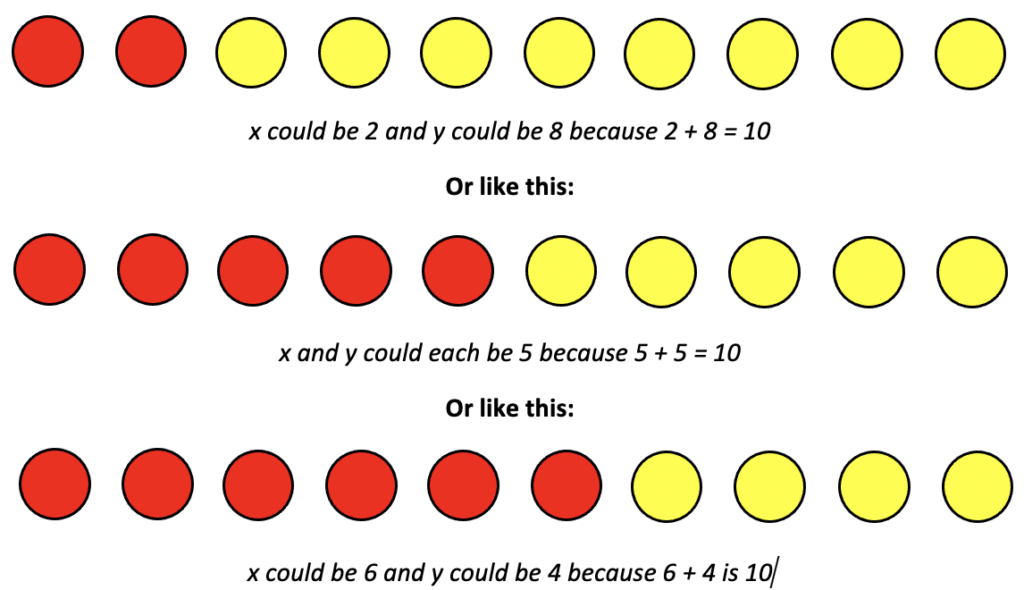
3. Reframe subtraction. One thing that makes it challenging to draw pictures of subtraction is that it often feels like an action – the action of taking away. However, a student who has developed operation sense with subtraction may be able to frame the first equation in one or more of the following ways:
- The difference between twice x and y is 11
- Twice x is 11 more than y
- If I add 11 to y, I’ll get the same number as if I double x.
How might these understandings make it easier to draw a picture or draw conclusions about the values of x and y?
4. Make logical inferences. What do we know about the numbers and their relationships? What conclusions can we draw? Because all the values in the answer choices are positive whole numbers, a student might come up with some inferences like these:
- x and y are both less than 10 because they add up to 10.
- 2x has to be more than y by 11, so x is probably the bigger number.
While it is always possible to approach a problem like this by testing out all the answer choices, taking a minute to think about the relationships and what numbers are likely to work may save time.
5. Make a table. Even if this weren’t a multiple-choice question, a quick table could help a student get a handle on what numbers would satisfy the system. Using the fact that the numbers have to add up to 10, a student might set up a table this way:
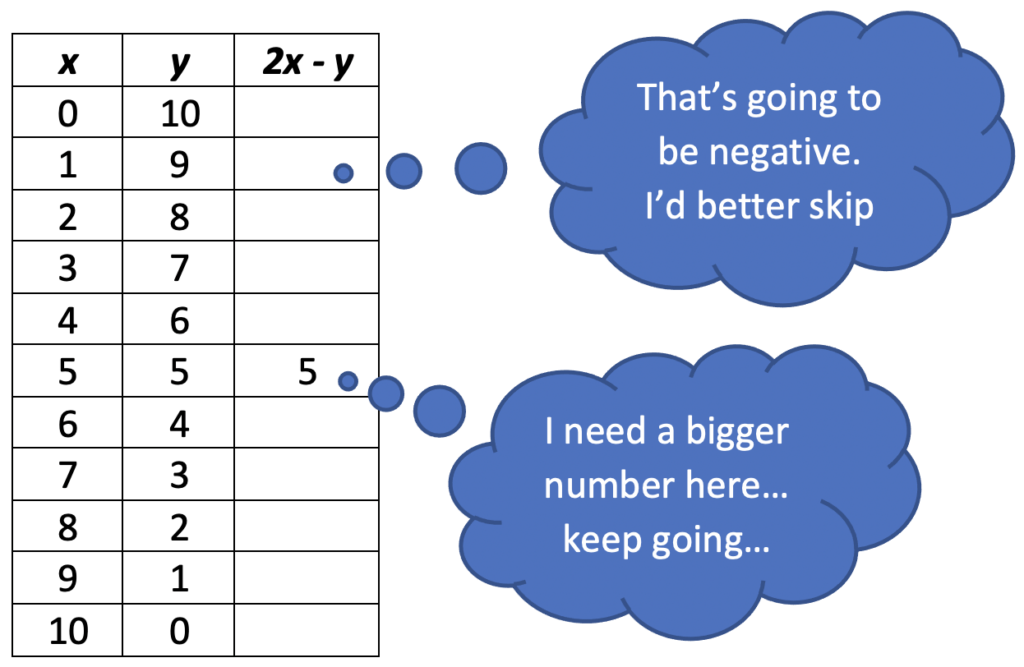
You may be wondering if this approach would be helpful if the answers were not positive whole numbers. The answer might not show up in the table, but it would still get you moving toward it.
Algebraic notation is a way of concisely recording relationships. It is a language, and languages are vehicles for ideas. The most valuable thing for students to learn about algebraic notation is that it tells a story and that they can make sense of that story. While there are procedures and patterns that can get you to solutions quickly and reliably, those are not the only ways to get to the solutions. And making the procedures and patterns the focus of math learning is like teaching students grammar and spelling without ever teaching the meanings of words or the ideas they communicate. This is not all to say that it is not worth teaching strategies like substitution and elimination, only that those are just some approaches – they are not the be-all and end-all to solving systems of equations. Students who learn and make sense of those procedures will find them to be powerful tools, but students who are empowered to see the stories in algebra can solve problems that they “haven’t learned how to do yet” if they approach them with confidence, flexibility, and a willingness to try different tools.
You may be wondering if this approach would be helpful if the answers were not positive whole numbers. The answer might not show up in the table, but it would still get you moving toward it.

Sarah Lonberg-Lew has been teaching and tutoring math in one form or another since college. She has worked with students ranging in age from 7 to 70, but currently focuses on adult basic education and high school equivalency. Sarah’s work with the SABES Mathematics and Adult Numeracy Curriculum & Instruction PD Center at TERC includes developing and facilitating trainings and assisting programs with curriculum development. She is the treasurer for the Adult Numeracy Network.
