Blog

Will This Be on the Test? (December 2023)

by Sarah Lonberg-Lew

---

Welcome to the latest installment of our monthly series, “Will This Be on the Test?” Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually.

---

Welcome back to our continuing exploration of how to bring real conceptual reasoning to questions students might encounter on a standardized test. Here is this month’s question:

When looking at textbooks, workbooks, and teaching software, it sometimes seems that the goal is to train students to automatically set up and solve a proportion using the cross product whenever they see the word “ratio.” We give students lots of practice solving for the missing number, but there is more to proportional reasoning than that. Here are some visual and conceptual strategies that a student who is reasoning about the question and about the context might use:

1. Make sense and estimate. An essential first step in any problem solving is making sense of the problem. It’s a step that both students and teachers sometimes skip in their hurry to get to a procedure that will produce a correct answer. A student who takes some time to think about the context and maybe restate the question in their own words will have a strong start and may even be able to use estimation to get an idea of which answer choices are more worth investigating. In this case, the idea of a ratio being “at least” 3:10 is a mathematically sophisticated and potentially confusing idea, but the context is very helpful. A simpler way of thinking about it is that it’s a question about whether there are enough nurses in a ward to take care of the patients there. Using approximate language to describe the relationships between the numbers of patients and nurses can be a good starting point. For example, a student might reason like this:

A) A ward with 5 nurses and 16 patients …. has a little more than 3 times as many patients as nurses.

B) A ward with 6 nurses and 18 patients … has exactly 3 times as many patients as nurses.

C) A ward with 8 nurses and 20 patients …. has between 2 and 3 times as many patients as nurses.

D) A ward with 10 nurses and 35 patients … has between 3 and 4 times as many patients as nurses.

E) A ward with 12 nurses and 40 patients … has between 3 and 4 times as many patients as nurses.

Based on this analysis, which ward or wards seem like they might not have enough nurses?

2. Draw out the ratios. A student can visualize the ratio in the ward and compare it to the desired ratio by drawing a picture of the nurses and patients, perhaps using letters to stand in for the people. For example, an illustration of the ward described in answer choice B (6 nurses and 18 patients) might look like this:

If we then draw loops around copies of the ratio in the hospital’s claim (making groups of 3 nurses and 10 patients), it might look like this:

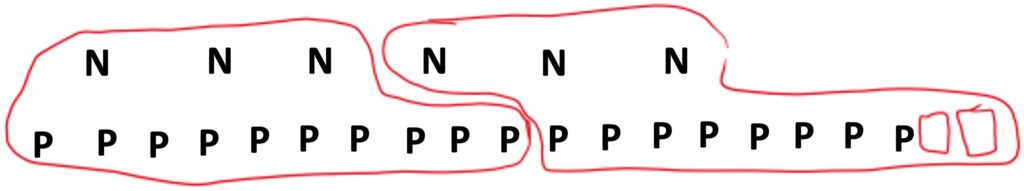
In drawing loops around groups of 3 nurses and 10 patients, the student might see that there are two patients “missing” from the second group or that there is room for two more patients in that group. What does this say about whether there are enough nurses? Try using this strategy to investigate some of the other answer choices.

3. Take advantage of friendly numbers. The staffing ratio the hospital is claiming to have is 3 nurses for every 10 patients. In answer choices C and E, the number of patients is a multiple of 10, so a student might ask how many nurses there should be in those wards and see how it compares to the number there are. For example, in answer choice C, there are 8 nurses and 20 patients. That’s two groups of 10 patients, so to have enough nurses, there should be two groups of 3 nurses or 6 nurses. Since there are 8 nurses in that ward, that is more than enough. How would you use that reasoning with answer choice E? How could you use similar reasoning looking for a friendly multiple of the number of nurses?

4. Get close and then reason about the leftovers. As in strategy 2, a student might chunk out what matches the claimed ratio and then look at what is left over. This can also be done without pictures. For example, a student might reason about answer choice D (10 nurses and 35 patients) by starting with a ratio table that gets close to the numbers and then exploring the leftover nurses and patients like this:

NURSES	PATIENTS
3	10
6	20
9	30

The ward described in answer choice D has 1 more nurse and 5 more patients than the last line in the ratio table. A ratio of 1:5 is not equivalent to a ratio of 3:10, but is it too many nurses or not enough? If 10 patients need 3 nurses, then 5 patients would need more than 1 nurse, so it is not enough nurses. (Important note: A strategy that does not involve drawing pictures is not better or worse than a strategy that does. The best strategy is what feels efficient and accessible to the person using it.)

Traditionally, when the topic at hand is proportional reasoning, or working with ratios, the end goal is to teach students to solve for the missing number in a proportion. However, in the real world, and even in test questions, there are other kinds of proportional reasoning to be done. In the case of evaluating whether a hospital ward has a reasonable staffing ratio (or for parents, a similar concern might be the teacher to student ratio at a school or daycare center), the question isn’t about exactly how many nurses there should be on the ward but about whether there are enough nurses on the ward for the number of patients. In this case, using a traditional method to find the exact number can give enough information to answer the question, but there are so many other ways to think about it, and often other approaches are more accessible and efficient. Which strategy is “the best” depends both on the numbers involved and the preferences and strengths of the person doing the reasoning!

---