Blog

Will This Be on the Test? (February 2021)

by Sarah Lonberg-Lew

Welcome to the latest installment of our monthly series, “Will This Be on the Test?” (You can find earlier installments as you scroll down the blog.) Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually.

I have heard an argument made that it’s not worth spending time teaching fractions in adult ed classes. One reason I’ve heard for this is that students are mostly in class to prepare for the test, and they can use a calculator on the test. Isn’t it more sensible and efficient to just spend one class period teaching them how to do fraction operations on a calculator and then moving on to higher level topics? After all, fractions are difficult and frustrating and it seems like you are always reteaching them anyway. Why not take the easy way out?

Unfortunately, taking the easy way out often doesn’t pay off in the long term – and in this case, it doesn’t pay off in the short term either. It doesn’t pay off in the long term because students miss out on real learning and sense-making that can help them make sense of the real-world math they will encounter. It doesn’t pay off in the short term because there’s more to solving test questions about fractions than just doing operations. High school equivalency tests are designed to test students’ mathematical reasoning, not their ability to use calculators.

Here is this month’s challenge. Imagine you are a student who is expert in doing fraction operations on the calculator but that is all you have learned about fractions. Would you be able to figure this out?

1.Estimate! The snail has been traveling for 3/4 of an hour already and is only going to travel for another 15 minutes (or 1/4 of an hour). Would it get a little further? A lot further? Twice as far? A quick sketch could help a student get a handle on what kind of answer might be reasonable:

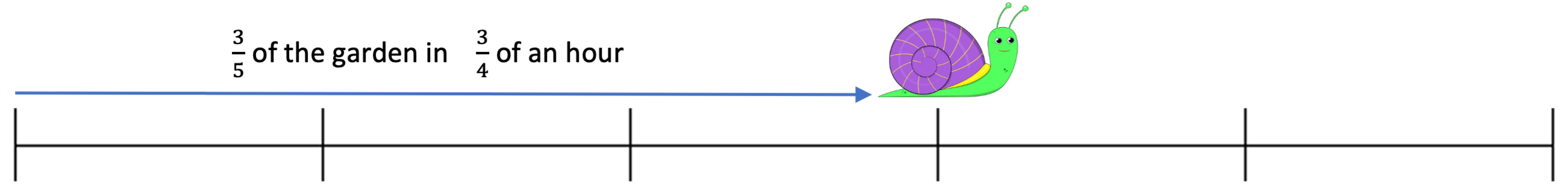
The snail is already more than halfway across the garden, so answer choices (a) and (b) don’t make sense because they are less than half. Answer choices (c) and (d) are both more than one, and it doesn’t look like the snail is going to make it past the end of the garden in only more 15 minutes, so those don’t make sense either. That leaves only one possible answer! (What fraction understandings were used here?)

2.Use a Singapore strip diagram. A student might start with a bar representing the 3/5 of the garden the snail has already crossed. Notice that it bears a resemblance to the sketch above: both show 3/5.

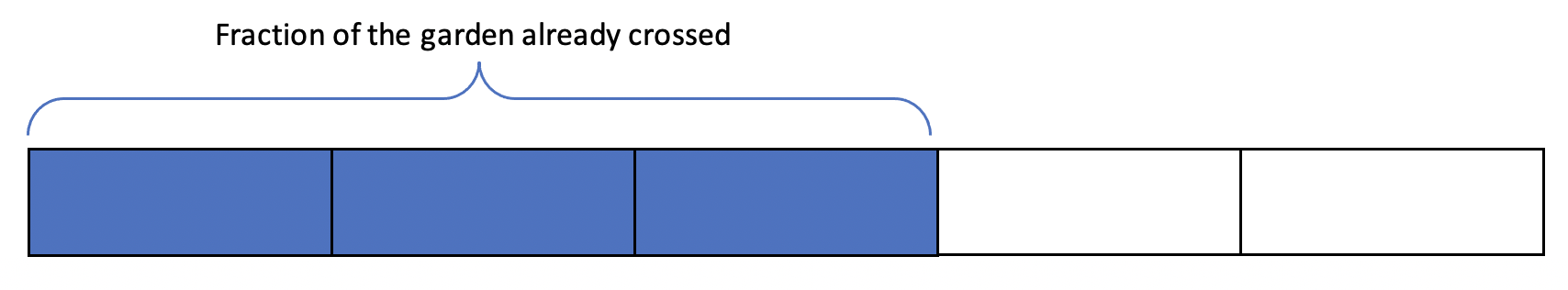
A student who understands that 3/4 of an hour means three groups of 1/4 of an hour, will be able to recognize that if the snail traverses three blocks in 3/4 of an hour, it is covering one block every 1/4 of an hour. (This is a non-trivial understanding and is important enough to have its own standard in the College and Career Readiness Standards for Adult Education: Understand a fraction a/b with a > 1 as a sum of fractions 1/b. (4.NF.3))

Filling in the fact that the snail covers one block every 1/4 of an hour will show the student the total fraction of the garden the snail will have covered after 15 more minutes:

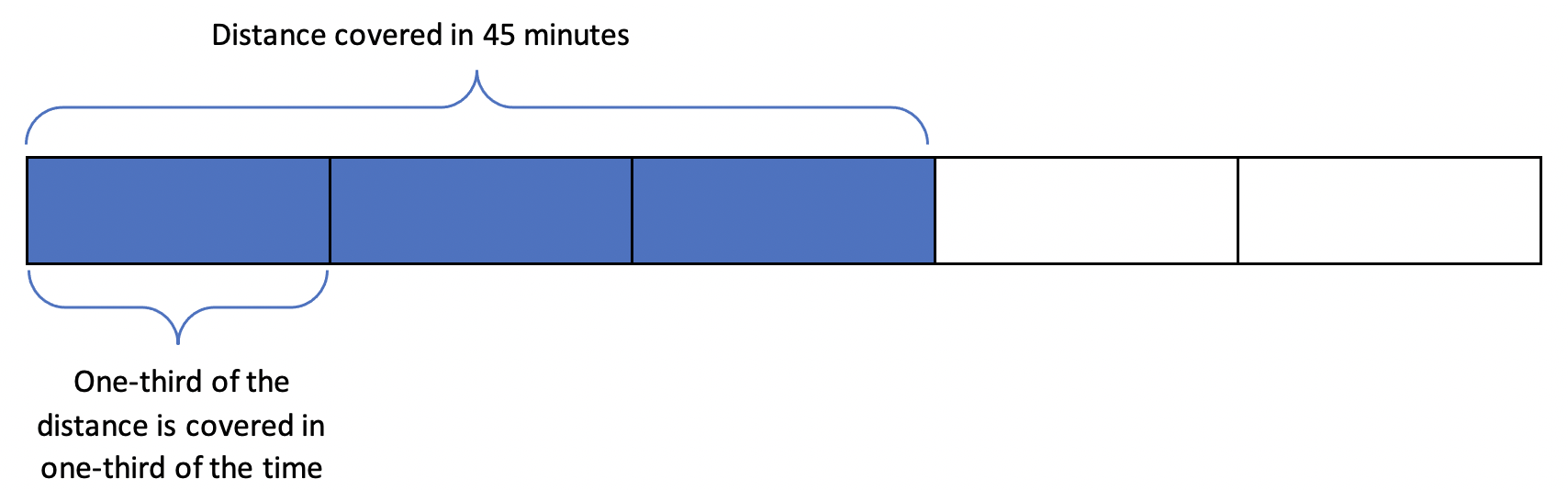
3.Reason proportionally. This task is about something moving at a constant rate. In other words, the distance the snail covers is proportional to the amount of time it has been traveling. A student might reason that 15 minutes is one-third of 45 minutes so the snail would cross an additional third of the distance it had already covered. A student who understands that 3/5 is three groups of  1/5 can recognize that 1/5 is one-third of  3/5,  so the snail will cover an additional 1/5 of the garden.

Did you notice that none of these approaches involved that old standard, “Choose and apply an operation”? Even after building a deep understanding of what is going on in this task, it may not be obvious which fraction operation, if any, is the ‘right’ one. It turns out that you can get the answer to this task using a fraction operation, so a calculator could be helpful to you if you can figure out which operation makes sense for the situation.

(Psst… want to know which operation it is? You can find the answer by dividing 3/5 by 3/4 because that is dividing distance by time which gives you a rate. Since the rate is in fractions of a garden per hour, the answer to this division gives you the total fraction traversed by the snail in one hour, which is what the question was asking for! This is also a good option, but the approaches explored above are probably more accessible to students.)

---